One of the greatest puzzles in theoretical physics is to find a consistent quantum theory of gravity, the only one of the four fundamental forces we do not know how to quantise yet. Although decades of intensive work, we have no conclusive answer to this problem. Currently one of the main contender in race towards quantum gravity is String Theory. It is based on a simple but far reaching idea, to substitute point-like particles with extended objects, strings and membranes. In this course, we will explore the implications of this idea. More precisely, we study the bosonic string and see that it not onl describes gravity but also gauge theories on D-branes. Gauge theories are required to describe the remainig three fundamental forces. This renders string theory to a theory of everything. The course is tailored towards master and PhD students who are familiar with

• electrodynamics
• special relativity
• quantum field theory.

Basic knowledge of general relativity is an advantage, but you also should be find if you did not have attended the GR course yet. There are many good books on the subject, four that I like are

• Barton Zwiebach: A First Course in String
• Blumenhagen, Lüst, Theisen: Basic Concepts of String Theory
• Polchinski: String Theory, Volume 1
• Johnson: D-Branes

Moreover, David Tong has some excellent lecture notes. We will have 2.5 hours of lectures and 2.5 hours of tutorial each week. Exercises will be posted here a week before the tutorial they are discussed in. Please keep in mind that active participation in the tutorials is important to pass the course. M.Sc. Luca Scala will be the assistant for the tutorials. Please fell free to contact him or me if you have any questions.

There are many other interesting approaches to Quantum Gravity and string theory is by far not the only one. There is another course this semester, Introduction to Quantum Gravity, which I highly recommend.

Important: Students will be assigned to exercise problems by the system described below, before the tutorial. Please familiarise yourself with this system and do not forget to indicate your preferences.

Additional material for the individual lectures, including the exercises which we discuss in the tutorials, will appear here in due time.

This is the tutorial for the course "Classical Field Theory" thaught by prof. Frydryszak for the Master in Theoretical Physics at the University of Wrocław. Exercises will be posted here a week before the tutorial they are discussed in. Please keep in mind that active participation in the tutorials is important to pass the course. M.Sc. Achilles Gitsis will be the assistant for the tutorials. Please fell free to contact him or me if you have any questions. For information about credits points, please refer to the syllabus (in Polish) or contact prof. Frydryszak.

Important: Students will be assigned to exercise problems by the system described here at the Friday, 9:00 pm, before the tutorial. Please indicate your preferences by then.

This is the mandatory Computational Methods I course of the Master in Theoretical Physics at the University of Wrocław. It is tailored towards master and PhD students who are familiar with

• computers and their operating systems (Linux is preferred, but Windows or MacOS will work, too)
• at least one programming language, ideally Python which we will use in the course
• classical and quantum mechanics.

There are many good books on the subject, four that I like are

• Landau , Páez, Bordeianu: Computational Physics: Problem Solving with Python
• Scherer: Computational Physics - Simulation of Classical and Quantum Systems
• Matthes: Python Crash Course - A Hands-On, Project-Based Introduction to Programming
• Johansson: Numerical Python

There will be 2 hours of lectures and 2 hours of labs each week. Exercises will be posted here a week before the lab they are discussed in. Please keep in mind that active participation in the labs and especially submitting the solution to the posed problems is important to pass the course. M.Sc. Biplab Mahato will be the assistant for this course. Please fell free to contact him () or me if you have any questions. For information about credits points, please refer to the syllabus or contact me directly.

Location: There has been a change of the lecture room. We are now in the computer lab 120 for both, the lectures and the labs.

Recordings/hybrid participation: The lectures will be streamed on YouTube with the link for each of them given below. This should help if you are not able to physically attend.

Exam: As discussed during the first lecture, we will have individual projects for the exam. If you have any project idea, we are very happy to hear about it and then decide together how to best implement it. Here are a couple of ideas which might help you

1. Molecular Dynamics Simulations: Simulate the behavior of a gas or liquid using molecular dynamics. For example implement the Lennard-Jones potential and study properties like diffusion, temperature, or phase transitions.
2. Quantum Mechanics Simulations: Solve the Schrödinger equation for a coherent state in the stadium billiard and compare its time evolution with the classical trajectory. Plot some of the eigenfunctions and discuss their properties.
3. Monte Carlo Methods: Implement a Monte Carlo simulation of the 2-dimensional Ising model or percolation. Explore random sampling techniques, convergence properties and/or phase transitions.
4. Astrophysical Simulations: which is either
   • N-body simulations or
   • solve the TOV equation
5. Electromagnetic Wave Propagation: Model the propagation of electromagnetic waves in various media. Implement the Finite-Difference Time-Domain (FDTD) method to study wave behavior, reflection, and refraction.
6. Chaos and Dynamical Systems: Explore chaotic systems, such as the logistic map or Lorenz attractor. Focus: Analyze stability, bifurcations, and strange attractors (other than the Lorenz attractor) using numerical methods.
7. Quantum Computing Simulations: Simulate simple quantum algorithms (e.g. Grover's, and Shor's algorithm or quantum teleportation). Implement quantum gates and circuits using Python with libraries such as Qiskit.

Once you have chosen a project and it is approved by us, you will prepare your Python code, a small documentation and some of the most important results and submit them to us by February the 3rd 2025 here on the website in the project section below. It is only possible to submit a single file. If you have multiple files in your project, please combine them to a ZIP-archive. After this, you will have a 15-minute presentation of your results during the exam session (dates and times are also given below) followed by 5 minutes of discussion. Projects will be presented on

• 12.02.2025 in room 426 and on
• 13.02.2025 in room 124.

Please find a list of all the projects and the respective dates and times below. The presentation part is public, therefore you are encourage to join at least some of the presentations of your peers.

For the last five tutorials, there will be no assignments. Instead you have the chance to discuss problems in the yet open assignment which you have to submit until January the 31st 2024 and challenges you encounter in your project.

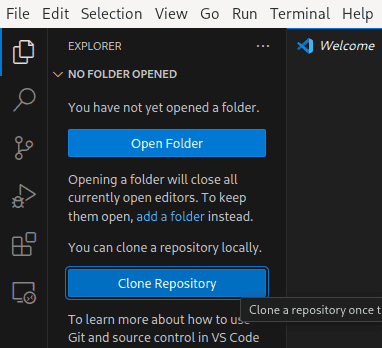
Development environment: In this course we will use the programming language Python with a notebook interface, called Jupyter notebooks. To get all this running, and avoid possible problems with incompatible versions of Python packages, we will use a standardized development environment. To set it up, please first install Visual Studio Code. On Linux, you will most likely have it already in your package manager. Alternatively, you can also download it here. Next, you need to clone the git repository for this course. To this end, open VS Code and click on the two documents (Explorer, Ctrl+Shift+E) to find something which looks like this

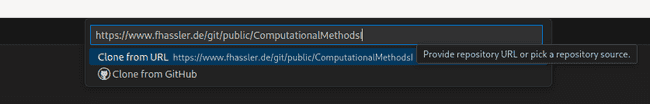
Now, click on "Clone Repository" and enter the URL of our git repository

https://www.fhassler.de/git/public/ComputationalMethodsI

like this

Finally you have to install all packages for the virtual environment, we use to run our Python code and notebooks. In the main menu, click on Terminal and then Run Build Task... (Ctrl+Shift+B). After this you should see some activity in the terminal on the bottom of the screen. If there are no errors, you have successfully installed the virtual environment. Now you are ready to add your own notebooks or run some of the already provided.

Additional material for the individual lectures, including the exercises which we discuss in the labs, is given below:

In this course, we will explore the holographic principle, stating that a volume of space can be equivalently described by its lower-dimensional boundary. One might think of a hologram which captures a three-dimensional object on a two-dimensional surface. A particular well studied realization of this idea is the AdS/CFT correspondence which conjectures the equivalence between quantum gravity in Anti-de Sitter (AdS) space and a conformal field theory (CFT) on its boundary. This conjecture is very hard to prove because when one of the two theories is weakly coupled, allowing to use perturbation theory to study it, the other one is strongly coupled - making it very hard to analyse. Despite still being a conjecture, this idea had a huge impact on high-energy physics with an extremely broad range of applications. Latter range from new insights into strongly coupled quantum field theory, required for example to study extreme states of matter like quark-gluon-plasmas or superconductors, to the analysis of black-hole evaporation, that ultimately requires a handle on quantum gravity. We take this as a motivation to study this duality and its main actors in this course in detail. The lectures are tailored towards master and PhD students who are at least familiar with

• electrodynamics
• special relativity
• quantum mechanics.

Basic knowledge of general relativity and quantum field theory is definitely an advantage, but we review their most salient aspects in case you have not yet attended a specialized course on them yet. We will mostly follow the book

• Martin Ammon, Johanna Erdmenger: Gauge/Gravity Duality,

but you might also want to have a look at

• Lewis Ryder: Introduction to General Relativity
• Michael Peskin, Daniel Schroeder: Quantum Field Theory
• Barton Zwiebach: String Theory

for more details on the main actors of the gauge gravity duality.

We will have 2 hours of lectures and 2 hours of tutorial each week. Exercises will be posted here a week before the tutorial they are discussed in. Please keep in mind that active participation in the tutorials is important to pass the course. M.Sc. Luca Scala will be the assistant for the tutorials. Please fell free to contact him or me if you have any questions.

Important: Students will be assigned to exercise problems by the system described here, before the tutorial. Please familiarise yourself with this system and do not forget to indicate your preferences.

Exam: We decided to have an oral exam (not longer than 45 minutes) for this course in the afternoon of Tuesday, the 11th of February 2025. The exact time will be individually assigned. To help you prepare, here is a preliminary list of relevant topics:

1. Anti-de Sitter space
   • isometries, and the corresponding isometry group
   • construction as hypersurface embeed into $\mathbb{R}^{d,2}$
   • bounday and its properties
   • different coordinate systems
2. Large N limit of SU(N) (super) Yang-Mills theory
   • planar diagrams
   • relation of the partition function to the string partition function
3. Conformal symmetry and supersymmetry
   • Coleman-Mandula theorem
   • algebras
   • representations
   • resulting simplifications in QFT calcuations
   • anomalies
4. String theory basics
   • specturm of open and closed strings
   • string perturbation theory
   • super strings and GSO projection
   • D-branes from the world-volume perspective (DBI action) and from the target-space perspective (black black solution)
5. AdS CFT correspondence
   • near horizon limit for a stack of N D3 branes
   • strong and weak for of the duality
   • field-operator correspondence
   • computing CFT correlation functions from supergravity solutions
   • holographic renoramlization

Retake Exam: If required, the retake exam can be taken on the afternoon of Tuesday, the 18th of February 2025. The same conditions as for the exam apply.

Additional material for the individual lectures, including the exercises which we discuss in the tutorials, will appear here in due time.

Lie algebras describe infinitesimal symmetries of physical systems. Therefore, they and their representation theory are extensively used in physics, most notably in quantum mechanics and particle physics. This course introduces semi-simple Lie algebras and the associated Lie groups for physicists. We discuss the essential tools, like the root and weight system, to efficiently work with them and their representations. As on explicit application of the mathematical framework, we discuss Grand Unified Theories (GUT). Moreover, we show how modern computer algebra tools like LieART can significantly help in all explicit computations throughout the course.

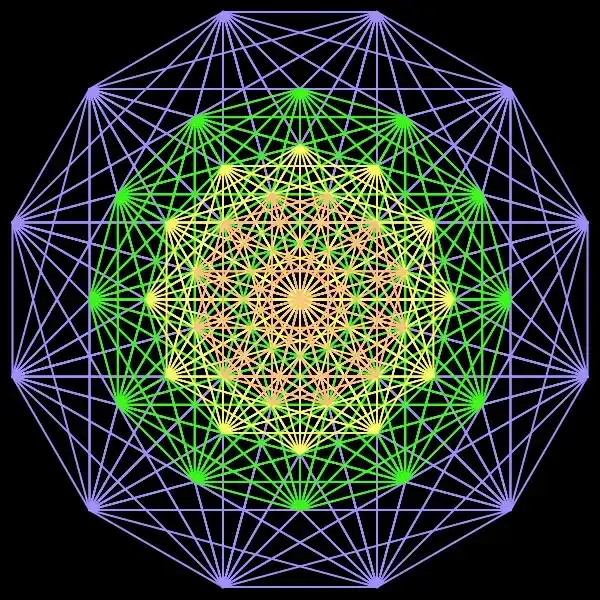A simple example is the visualisation of the root system of $E_6$ projected on the Coxeter plane, which you can see here. If you want to understand how it is created and connected to particle physics, you should take this course. Basic knowledge of core concepts in linear algebra, like vector spaces, eigenvalues and eigenvectors, is assumed. Some good books about the topic are:

• Fuchs and Schweigert: Symmetries, Lie Algebras and Representations
• Gilmore: Lie Groups, Lie Algebras, and Some of Their Applications
• Fulton and Harris: Representation Theory
• Georgi: Lie Algebras In Particle Physics: from Isospin To Unified Theories

The article Phys. Rep. 79 (1981) 1 by Slansky and the manual of the LieART package are good references, too.

Note: At multiple occasions, we will use Mathematica and it might be a good idea to set it up on your computer. Following the instructions on the main teaching website, you should be eventually able to run the notebook, which generates the projection of the $E_6$ root system above.

Exam: After a majority vote, we decided together that the written exam for this course will take place on Tuesday, the 6th of February 2024, at 13:00 in room 447 (the one where we also have our classes). It will take two hours and you have a practise exam to help you preparing for it. Please be there five minutes earlier such that we can start on time.

Retake Exam: As discussed via email, you have the chance to take part in the retake exam on Thursday, the 15th of February 2024, at 13:00 in room 447. Exactly the same conditions as described for the exam above will apply. If you would like to take part in the retake exam, please send me a quick message via email such that I have an estimated head count and can bring the right number of exams.

This is the mandatory Quantum Field Theory course of the Master in Theoretical Physics at the University of Wrocław. It is tailored towards master and PhD students who are familiar with

• quantum mechanics
• electrodynamics
• special relativity
• quantum electrodynamics.

There are many good books on the subject, four that I like are

• [PeskSchr] Peskin, Schroeder: An introduction to quantum field theory
• [Ryder] Ryder: Quantum Field Theory
• Weinberg: The Quantum Theory of Fields, Volume 1 & 2
• Zee: Quantum Field Theory in a Nutshell.

We will follow mostly the first one in the lectures. There will be 2 hours of lectures and 2 hours of tutorial each week. Exercises will be posted here a week before the tutorial they are discussed in. Please keep in mind that active participation in the tutorials is important to pass the course. M.Sc. Biplab Mahato will be the assistant for the tutorials. Please fell free to contact him () or me if you have any questions. For information about credits points, please refer to the syllabus or contact me directly.

Important: Students will be assigned to exercise problems by the system described here at the Thursday, 9:00 pm, before the tutorial. Please indicate your preferences by then.

Exam: After a majority vote, we decided together that the written exam for this course will take place on Monday, the 3rd of July 2023, at 14:00 in room 447 (the one where we also have our classes). It will take two hours and we will discuss a practise exam in the last tutorial to help you preparing for it. Please be there five minutes earlier such that we can start on time. Also note that you need to have more than 50% of the points from the exercises assigned to you to qualify for the exam. You can check your points here on the website, or, if you are in doubt, with me.

Retake Exam: As discussed via email, you have the chance to take part in the retake exam on Friday, the 8th of September 2023, at 17:00 in room 447. Exactly the same conditions as described for the exam above will apply. If you would like to take part in the retake exam, please send me a quick message via email such that I have an estimated head count and can bring the right number of exams.

Additional material for the individual lectures, including the exercises which we discuss in the tutorials, is given below: